Properties in Terms of True Stress and Strain

Under some circumstance, particularly when the material is in plastic range of deformation, certain material properties are defined in terms of true stress and strain. True stress plotted against true stain is best represented as in Figure 14(a) with properly choosing strain scale because it is in plastic range. In most cases the relationship is non-linear and the slope of the curve at any given strain is called modulus of strain hardening at that strain.

Several experimental observations recommend that σ′ and ε′ satisfy following relation

                   σ′ = k ε′n                                         ---------- (1.16)

or  

               ln σ′ = ln k + n ln ε′.                             ----------(1.17)

 
where, k and n for a given material can be regarded as its properties, k is called strength coefficient while n is strain hardening exponent. As is obvious from Eq. (17) the plot between ln σ′ and ln ε′ is a straight line whose slope is equal ton and intercept on ln σ′ axis is ln k.

The constants k and n can be determined experimentally if diameter of specimen corresponding to load P is recoded. A special gauge will have to be used for measuring least diameter along gauge length with increasing load.

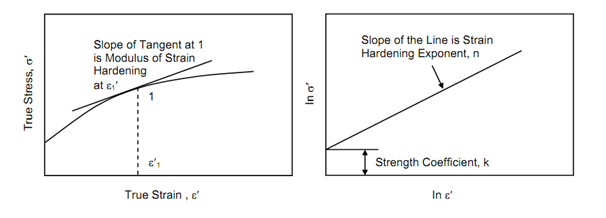
      Figure: True Stress Plotted as Function of True Strain (a) Linear Scale; and (b) In Scale

One may refer to Figure 2 and once more may note that at point d the stress is maximum and even when strain keeps increasing stress σ keeps reducing after point d. in an experiment wherein a ductile material is being tested perceptible reduction in diameter is observed after ultimate point, d. The condition of beginning of reduction of diameter in the localised region is termed necking and it is the neck where diameter is always minimum. This phenomenon is also called instability and cannot be reversed. However, if load is removed very fast further necking may be stopped. If the load is maintained then necking will continue until fracture.
It is possible to establish the condition of beginning of necking in terms of strain hardening exponent. Starting from the fact that tangent to load elongation curve at highest point will be horizontal, it can be written that

                                                           dP = 0 or,   

                                                          d (σ′ A) = 0
where, σ′ is true stress and A is the area of cross-section of tension specimen at maximum load.

∴                                                   σ′ dA + A d σ′ = 0

                                                  or    d σ′ / σ′=− dA/ A                                    ---------- (18)

Also since volume of specimen of length L and cross-section area A remains constant, that means AL = constant.

                                                        d (AL) = 0

                                              or,    L dA + A dL = 0
i.e.

                                                 − dA/ A = dL/ L = d ε′                                     ----------- (19)

 From Eqs. (18) and (19)

                                                     d σ′ / σ′     = d ε′

                                                    or,    d σ′ / d ε′ = σ′                              ----------- (20)

Substitute σ′ from Eq. (16) in Eq. (20) to obtain

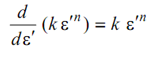
i.e.
                                                  n k ε′^{n −}1  = k ε′ⁿ
or,
                                                    ε′ = n                                            ---------(21)

Thus it is seen that when true strain ε′ becomes equal to strain hardening exponent n, necking will begin and fracture will ultimately occur. Metal working process like drawing, rolling, extrusion etc. may be thus performed safely it at no stage the true strain is allowed to reach the value of strain hardening exponent. The strain hardening exponent can be determined from tension test. Such determination will be exemplified in solved example.