Q:  Calculate the value of voltage V_o from Thevenin's theorem.

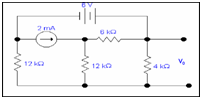
Solution:

            We have to calculate V₀ by using Thevenin's theorem .

Step 1:      Remove R_L

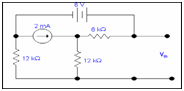
 In the circuit value of R_L is equal to 4k resistor at which we have to calculate the voltage V_o.

Step 2:  Calculating the value of V_th

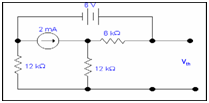
We have to calculate V_th .By applying KVL in two loops to compute the individual loop currents.

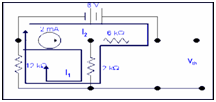
                                    Here, I₁ = 2mA

                        By applying KVL for the super mesh

                                    -6 +6kI₂ +12k (I₁ +I₂) +12k (I₁ +I₂) =0

                                         -6 +6kI₂ +12kI₂ +24kI₁ + 12kI₂ =0

                                                          30kI₂ - 6 +24k (2m) =0

                                                30KI₂ -6 +48 =0

                                                I₂ = -42/30mA

                                                I₂= -1.4mA

Now calculate the voltage around 6k & 12 k to estimate the value of V_th

                        So                     V_6k = 6kI₂

                                                = 6k(-1.4m)       //putting the value of  I₂

                                                V_6k = -8.4V

                                                V_12k = 12k (I₁ +I₂)

                                                        = 12k( 2m -1.4m)  =7.2V 

                        Therefore,

                                                V_th = V_6k + V_12k

                                                                        = -8.4 +7.2 = -1.2V

Step 3:  Determining value of R_th

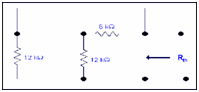
            6k is in series to 12k .The resultant of these will be in parallel with 12k.

                                    So,       (6k+12k) ||12k = 18k x12k/ (12k+18k)

                                                             R_th = 7.2k

Step 4: Determining the unknown quantity.

After computing V_th & R_th, re-inserting the load resistance R_L in the circuit in series along R_th and supposing the V_th like a battery in series along these two resistances.

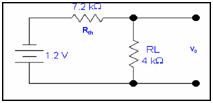
                                          V₀ = -1.2 x4k/(7.2k + 4k)

                                         V₀ = - 0 .4285V