Find out the load carried by each of cylinder:

A hollow steel cylinder of cross-sectional area 2000 mm² concentrically surrounds a solid aluminium cylinder of cross-sectional area 6000 mm2. Both cylinders contain the similar length of 500 mm before a rigid block weighing 200 kN is applied at 20^oC as illustrated in Figure. Find out

(a) the load carried by each of cylinder at 60^oC.

(b) the temperature rise needed for the total load to be carried by the aluminium cylinder alone.

        Figure

For computation purposes, take following values :

E_steel = 210 GN/m2 and E_aluminium = 70 GN/m²

σ_steel = 12 × 10^{- 6} K^{- 1} and α_aluminium = 23 × 10^{- 6} K^{- 1}

Solution

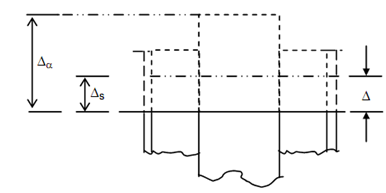
Below given Figure shows the free thermal expansions Δa & Δs together with the common expansion Δ under the load of 200 kN (the subscripts a & s standing for aluminium & steel respectively).

For a temperature grow up of ΔT K,

We get,

Δa = 500 × 23 × 10^{- 6}  × ΔT = 11.5 × 10^{- 3}  ΔT mm

Δs  = 500 × 12 × 10^{- 6}  × ΔT = 6 × 10^{- 3}  ΔT mm

                                    Figure

Under load, the strains are

ε _α  = (Δ_a  -Δ )/ 500

and

ε_σ  =   Δ _s -Δ /500

and the equivalent stresses are as follows :

σ_a  =     (70 × 10³)/ 500  (Δ_a  - Δ) = 140 (Δ_a  - Δ) N mm^-3

σ_s  =( 210 × 10³)/500   (Δ_a - Δ) = 420 (Δ_a - Δ) N mm^{- 2}

For equilibrium of vertical forces,

σ_a  × 6000 + σ_s  × 2000 = 200 × 10³  N

putting for σ_a, σ_s, Δ_a and Δs, we obtain

(11.5 × 10^{- 3 ×} ΔT - Δ) + (6 × 10^{- 3}  ΔT - Δ) =  5 / 21

 Hence,

 Δ= 8.75 × 10^{- 3}  ΔT -  (5 /42)

 The loads taken by the aluminium & the steel are thus,

P_a  = σ_a  × 6000 N

= 840( 2.75 × 10^{- 3}  ΔT + (5/42)  kN

P_s  = σ_s  × 2000 N

= 840 (   (5/42) - 2.75 × 10^{- 3}  ΔT ) kN

These equations shall be valid as long as Δ is less than Δs. the load shall be totally carried by aluminium when Δs becomes equal to Δ.

(a)        at 60^oC,

ΔT = 60 - 20 = 40 K

P  = 840( 2.75 × 40 × 10^{- 3}  +( 5 / 42))

= 192.4 kN

P_s  = 200 - 192.4 = 7.6 kN

 (b)       The load shall be carried totally by aluminium while

6 × 10^{- 3} × ΔT = 8.75 × 10^{- 3} × ΔT - ( 5/42)

or         ΔT = (5 × 10³) / (2.75 × 42)

= 43.3^o C

That means at a temperature of (20 + 43.3) = 63.3^oC.