Strain Energy

A load applied at any point of a body will reasons deformation and the point of application of force will move. The condition satisfies definition and several works would be done. Such work is stored in the body as strain energy. Consideration of definition in a complex system of forces would be difficult. Thus a simple example of bar of uniform cross-section, held rigidly at the upper end and at whose lower and a slowly or gradually increasing load P is applied, is considered. In given diagram (b) a plot of load P and extension d is shown, and it can be seen that this plot is linear up to specific load P₁ and then is curved. P₁ or stress corresponding to P₁ that is P₁/A = σ₁ may be regarded like limit of proportionality. Limit of proportionality is lower than elastic limit. Given diagram(c) depicts a plot among strain and stress of the bar and is essentially similar in nature as like depicts a plot between stress and strain of the bar and is essentially same in nature as P-δ plot since σ(= P₁/A) and ε (=δ/L)  represent  load and extension on different scales.

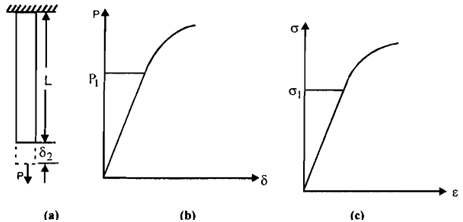
Diagram of (a) A Bar Held Rigidly at Top; (b) Load-extension Diagram; and (c) Stress Strain Curve .Then the work done will be, if the deformation is recoverable elastic

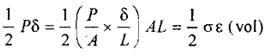
For deformation beyond elastic limit it can be displayed that work done per unit volume is:

∫σdε.

Hence by definition strain energy per unit volume is ∫σde or ½(σe) within elastic limit. Such elastic strain energy is denoted by U and using eqn 17, one can write:

                                                      U = (AL) σ²/2E = ((AL)/2)Eε²............................................eqn21

The quantity σ²/2E is often referred to as strain energy density and is equivalent to the area under the stress-strain curve. The under σ-e curve up to any value of strain that is beyond elastic limit such will  still represent the strain energy density but will not be described by eqn 23 in previous section because eqn 17  is not true for inelastic deformation.

For loads in the elastic limit the stress-strain curve on unloading coincides along with the loading line. Thus all the work put into the body in deforming it is recoverable upon release of load. The maximum energy per unit volume such can be absorbed with no causing inelastic deformation is termed resilience's modulus.

If we define yield strength σ_g as criterion for failure in tension test after that the energy at failure.  In any body of any shape and subjected to any stress combination such will be equal to

                                                    µ = (1/2E)σ²_g per unit volume.......................................eqn22

It will be considered later.