Shear stress distribution in cross sections:

Consider the width of beam at the level EF be b.

At the level EF, the intensity of the horizontal shear stress is τ.

 Then,

τ =  Unbalanced force / Shear area

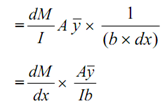
As we have seen earlier, dM/ dx = F, the shear force, and shear stress, 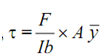Where A is refer to the area of cross section of the beam above the level EF, & 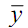is the distance of the centroid of the area above the level EF, through the neutral axis.

For a specific cross section of a beam, F remains constant for particular point along the depth of the beam. Thus, we can conclude that the shear stress at any point on a cross section is proportional to 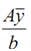For sections of uniform width, the shear stress shall have maximum value at the neutral axis, since 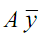is maximum at the neutral axis. The shear stress shall have zero value at top & bottom layers of the beam cross-section, As  is zero at these layers.