Risk Transfer:

Consider a logarithmic utility function which exhibits declining absolute risk aversion. So more wealthier you are, the  lower is your cost of bearing a fixed monetary amount of risk. When utility cost of risk is declining in wealth, less wealthy people could pay more wealthy people to bear the risk. Consequently, both parties would be better off.

Example

The utility function is represented as u(w) =  ln(w). Let an individual face a 50 percent chance of  losing Rs.100.  The willingness of  this person to  pay  for eliminating this risk depends on her initial wealth.  

If we assume the initial wealth is Rs.200,  the expected utility can be obtained as

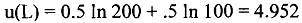
The  certainty equivalent of  this lottery  is  exp(4.59) = 141.5. Therefore, the individual would be willing to pay Rs.8.50 to defray this risk. Let there be an individual with initial wealth of Rs. 1,000. We assume that the utility function is same with that of the above case. Then the expected utility

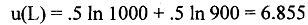
The certainty equivalent  of  this lottery  is  exp(6.855)  = 948.6. Hence,  this agent would  be  willing to  pay  only  Rs.1.4  to defray the risk. See that  the wealthy rnemb-r could fully insure !he  poor member at a cost of Rs.  1.4 while the poor member would be willing to pay Rs.8.5 for this insurance. Therefore, they may  agree for a price between Rs.1.4 and Rs. 8.5. Such an outcome will be Pareto improvement.