Find out by the real roots of the denominator:

The singularities, corresponding to the points at infinity of C (u) , are find out by the real roots of the denominator

ω(u) = (1 - u)²  + 2u (1 - u) ω₁ + u ²  = 0

They are given by

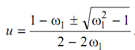
The curve reduces to

(i)                 a straight line if space w₁ = 0

(ii)               an elliptic segment if 0 < w₁ <1

(iii)             a parabolic segment if w₁ = 1

(iv)              a hyperbolic segment if w₁ > 1

Geometrically, the curves are governed by the Parameter s = ω₁/1 + ω₁ as

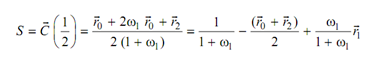
The curvepasses through 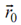(= P₀ ) and 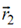(= P₂ ) along with the slope from

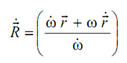
Therefore

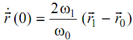
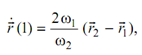  , the tangents meets at  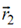 (= P )