Principal planes for the state of stress:

Appraise the principal stresses & principal planes for the state of stress illustrated in Figure.

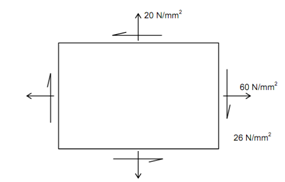
     Figure

Solution

Given σ_x = 60 N/mm²

σ_y = 20 N/mm²

t_xy = - 26 N/mm²

By putting in Eq. , we obtain

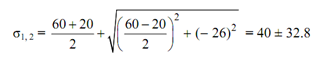
∴          σ₁ = 72.8 N/mm² and  σ₂ = 7.2 N/mm²

Again putting the values σ_x, σ_y and σ_xy in Eq.

 tan 2f = 2t _xy / (s _x - s _y)

 =  2 ´  (- 26) / (60 - 20)

= - 1.3

Since q is general angle, the specific angles representing the principal planes are designated as f₁ and f₂.

∴ 2f = - 52.43º, 127.57º

By using          2f = - 52.43º

 σ_n = (60 + 20)/2 +( ( 60 - 20)/2 )cos (- 52.43º) - 26 sin (- 52.43º)

= 72.8 N/mm²

Hence, we recognize that f1 =- 52.43^o / 2 describe the major principal plane and then, f₂ =127.57^o / 2 must define the minor principal plane.