Modulus Of Elasticity Of A Composite
For establishing a method of calculating effective modulus of elasticity let us consider a simple case of a longitudinal composite as shown in Figure. The composite is made of two constituent – a matrix M and a fibre F. Let the length of both be unity. While their cross-sectional areas are respectively Am and Af so that the volume of matrix is Am and that of fibre is Af. Let total force P act axially and force carried by fibre be Pf and that carried by matrix be Pm so that
Pf + Pm = P ----------------- (1)
Remember that the condition for perfect adhesion between the fibre and matrix is that both will extend by the same amount so that there is no relative displacement between the fibre and matrix. If modulus of elasticity of fibres is Ef and that of matrix is Em then their individual elongations can be calculated.
δ = Pf / A f E f and δ = Pm / Am Em ----------- (2)
And note that
δ f = δm ------------ (3)
Figure: Longitudinal Composite
If the composite is regarded as single body subjected to a force P, its cross-sectional area will be ( A f + Am ) and if its modulus of elasticity is E, then elongation,
δ= P /( A f + Am ) E
However, δ= δ f = δm
Hence from Eq. (3),
E = ( A f + Am ) δ= E f A f δ+ Em Am δ
or,
E = E f V f + Em Vm -------------- (4)
Note,
Vm = Volume of matrix,
Vf = Volume of fibre,
V = Volume of composite,
vm / V = Am / A f + Am = Vm = Volume fraction of matrix, and
vf / V = A f / A f + Am = V f = Volume fraction of fibre.
To understand the fundamental requirement of a composite in terms of its constituents Eq. (1) is quite helpful. For instance, let a composite made of polyvinyl chloride and glass fibres for which moduli of elasticity are respectively Em = 350 MPa and Ef = 50,000 MPa. Also remember that Vf = (1 – Vm) then for composite. E = 70000 (1 − Vm ) + 350 Vm = 70000 − 69650 Vm
Following values of E can be obtained for different values of Vm.
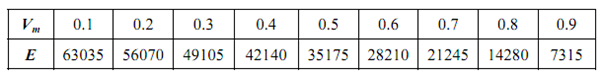
It can be noted that with decreasing volume fraction of weaker matrix the modulus of elasticity of composite increases. Naturally, the cost of this composite will approach that of the fibres as its volume fraction will also increase with decreasing fraction of matrix. However, this analysis also indicates that in a composite a desired property can be produced and this convenience is an added advantage.
It may also be noted that from the condition of load sharing between fibre and matrix we can write that
σc ( A f + Am ) = σ f A f + σm Am
where, σ stands for stress and suffixes, c, f and m indicates composite, fibre and matrix respectively. Area of cross-section of composite is (Af + Am). Apparently (A f / A f + Am ) = V f and ( Af / Am + Am ) = Vm
σc = σ f V f + σm Vm ------------ (5)
This equation gives method of calculating the permissible stress in composite. The basis of deriving both Equation (4) and (5) is the supposition that load on the composite is applied along the direction of fibres. It is also supposed that the bond among fibres and matrix is perfect and no slip takes place nor any fibre is broken. The situation under which strains in composite, fibres and matrix have been supposed to be same is called isostrain.
The modulus of elasticity in addition to stress carrying capacity of such a composite shall be drastically decreased if load is applied in a direction perpendicular to length of fibre. To achieve strength in two perpendicular directions the fibres might be placed in the matrix along two perpendicular directions. Though, calculations of both the modulus & stress in the composite in this case shall not be so simple.