Common cross-sections:

It is much more complicated to analyse non-circular cross-sections for plastic deformation. Theory of elasticity provides the solution with different types of materials which can show different τ-γ relationships. We will avoid that analysis.

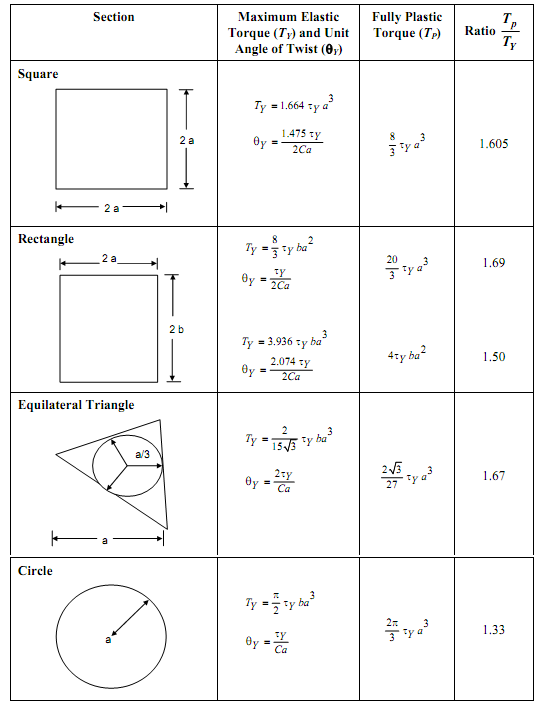
However, fully plastic torques for a few common cross-sections is listed in Table and is compared with maximum elastic solutions for these cross-sections. Expressions for fully plastic torques for a number of common structural sections are listed in Table.

In the calculations of the fully plastic torque for any hollow torsion member, the method of analysis is same to that for elastic torsion of the hollow torsion member, since the stress function φ (x, y) is flat-topped (has zero slope) over the hollow region of the torsion member. In order to simplify the analysis, just hollow torsion members of constant wall thickness are considered. For as torsion members, the fully plastic torque, T_p is obtained by subtraction from the plastic torque, T_ps of any solid torsion member with the boundary of the outer cross-section, fully plastic torque, T_pH of a solid torsion member having a cross-section identical to the hollow region.

             Table : θ_Y, T_Y, T_p  and     T_Y/ T_p  for Some Common Cross-sections