Inverse Elasticity Rule:
The Inverse Elasticity Rule (IER) is often used in policy making as an approximation for equal percentage change. Let us assume that demands for different goods are unrelated, i.e., cross price elasticity of demand are zero along with zero income effects. Under certain conditions, Ramsey rule simplifies to the 'Inverse Elasticity Rule': the tax rate is inversely proportional to a good's own compensated elasticity of demand. Taxes should in fact vary across the commodities such that goods with lower elasticity attract higher tax rates, as the extent of price change is smaller than goods with higher elasticity. In order to minimise substitution effect, elastic goods should be subject to lower tax rates and vice versa to keep distortions and, thereby, deadweight losses at minimum as mentioned earlier. The exemption of grocery from taxes is an example, which is counter to the basic Ramsey rule because grocery demand is relatively inelastic. But in order to make sales tax less regressive, these items are generally exempt for equity reasons.
Tax as a proportion of consumer price is inversely related to the price elasticity of demand. The Rule can be expressed as follows,
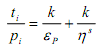
Where ti is the per unit tax on commodity (or factor) i, pi is the post-tax price, εP is the compensated price elasticity of demand, çs is the elasticity of supply, and k is a proportionality factor that depends on the total amount of revenue that the government seeks to raise. Assume that elasticity of supply is infinite (a horizontal supply schedule) so that (k/çs ) tends to become zero.
Recall that the extent of change in price-quantity equilibrium configuration depends also on the slope of the supply schedule. Therefore, assuming supply elasticity to be infinite, higher the price elasticity, lower should be the tax rate, hence the Inverse Elasticity Rule. As a consequence, taxpayers would tend to move away from the highly taxed commodities to the lightly taxed
commodities.
For two goods i and j, Ramsey rule can be expressed as follows. Let rates of tax t i and t j be levied on i and j. Taxation is efficient when the combination of t i and t j minimises the combined excess burden in the two markets i and j subject to collection
of a given amount of revenue, say R. Efficient tax rates of ti and tj therefore minimise the sum of excess burden in the two markets i and j as follows, Minimisation of (Excess burden in i + Excess burden in j) subject to fulfilment of the revenue target of the government R = tiPiQi + tjPjQj where, Qi and Qj are the quantities of i and j respectively sold in the market at prices Pi and Pj respectively.
Market structure is assumed to be competitive and supply is at constant costs. The above problem yields the following expression,
How do we determine the pattern of taxes such that deadweight loss is minimum? Optimality requires that the tax rates should be determined in a manner such that increase in deadweight loss per extra rupee collected is the same for each good (or factor). If the equality is not maintained, there exists a possibility of changing the tax rate so as to bring down the extent of deadweight losses by moving away from the good with higher marginal deadweight loss to the lower one until equality is restored. Optimal Commodity taxation: The marginal excess burden (deadweight loss) for marginal dollar raised must be the same for all commodites.
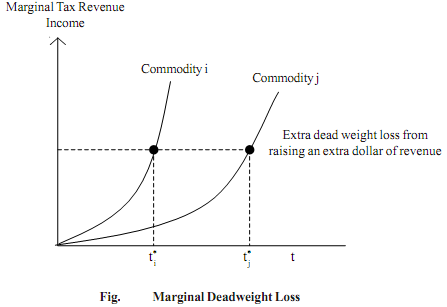
The figure depicts the solution to the optimal commodity tax problem. The ratio of the increase in deadweight loss to the tax revenues from raising the tax rate by a very small amount - that is marginal deadweight loss from raising an extra rupee from a tax on commodity i is computed. This has to be equally true for any other commodity, say j, as well.