Astable Multivibrator using Op-amp Comparator:

An astable multivibrator using an op-amp comparator is utilized to produce symmetrical square wave signal which is needed in many applications. For instance, if a square wave generator is available one may obtain triangular waveform by integrating square wave and from a triangular waveform, one may make a sinusoidal waveform by an appropriate wave shaping circuit such as, a triangular to sign wave converter. Therefore, a square wave generator can constitute an important building block of a function generator.

An astable multivibrator by using an op-amp comparator with positive feedback is illustrated in Figure, where op-amp along a resistors R_B and R_A may be seen to be configured as a Schmitt trigger. By analysis, it may be shown that this circuit may generate square wave whose time period may be set by proper selection of component values R, C, R_B and R_A.

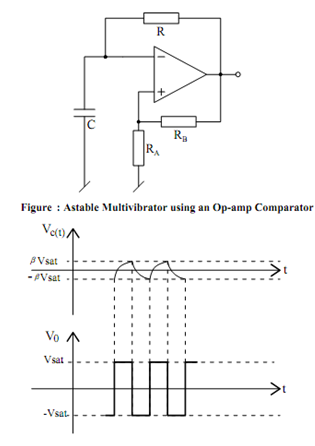
Figure : Various Waveforms for the Astable Multivibrator

For the analysis of this circuit, let us suppose that the output V₀ = + V_sat. Therefore , It is seen that the input signal at the non-inverting input of the op-amp comparator shall be + β V_sat, where β =   R_A / (R_A  + R_B) . Thus, the capacitor voltage V_c shall be charged exponentially towards + V_sat by time constant RC. V_c shall continue to grow until it becomes a little more than + β V_sat at which time the comparator shall switch to - V_sat.

The reference voltage at the non-inverting terminal shall now change to - β V_sat. As the capacitor voltage has already attained a value equal to + β V_sat it shall now try to discharge exponentially towards - V_sat. This shall continue until V_c attains a value equal to + β V_sat at which time the comparator shall again switch back to + V_sat. This procedure continues and the resulting wave forms of the capacitor voltage V_c and comparator output V₀ shall be as illustrated in Figure  It may be noted that since the charging and discharging, both are occur with the same RC network, the resulting square wave shall be having T_H = T_L. Also, the capacitor voltage shall be alternating between - β V_sat to + β V_sat while the output square wave shall be alternating between + V_sat and - V_sat.

The frequency of the output waveform may be calculated as follows. The general equation for V_c may be written as

                                                      V_c (t ) = A + B e ^{- t /RC}

where the constants A and B may be determined by using the following conditions : at

t = 0, V_c (t)= + β V_sat , at t = ∞, V_c (t) + V_sat  and for t = TH, Vc (t) = - β V_sat . Using these conditions, it is found that

                                              T _H = T _L = RC ln  [ (1 + β) /(1 - β) ]