Analytic and Geometric Properties:

The curve from Eq. (1) might be rewritten into the following corresponding form :

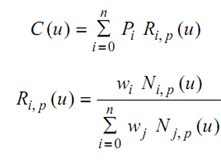   ---------------- (5)

here R_i, p (u) are rational basis functions. Their analytic properties find out the geometric behavior of curves. The most important properties are following :

- Generalization : If all of the weights are set to 1, then

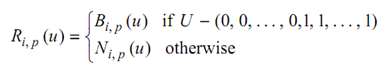
here the 0s & 1s in U are repeated along multiplicity p + 1, and B_i, p (u) mention the Bernstein polynomials degree.

-  Locality: R_i, p (u) = 0 if u ∉ [u_i , u_{i + p +1} ] .

-  Partition of Unity: ∑ R_{i, p} (u) = 1 .

-  Differentiability: In the interior of a knot span, the rational basis functions are infinitely constantly differentiable if the denominator is limited away from zero. At a knot they are p-k times constantly differentiable where k is the multiplicity of the knot.

                                  R_i, p (u; w_i  = 0) = 0

                                  R_i, p (u; w_i  →+ ∞) = 1

                                    R_i, p (u; w _j  → + ∞) = 0          j ≠ i