Chamberlin's Oligopoly Model:

Chamberlin suggested that a stable equilibrium can be reached in an oligopolistic market if the firms charge monopoly price. This will be possible if the firms recognise their interdependence, unlike in the Cournot model where they act on the naïve assumption of rival marinating its previous period's price or output level.

In this model, the setting (i.e., assumption) is similar as that in Cournot's except for the fact that the firms do recognise their mutual interdependence. Let us study the model on the basis of the following diagram. Let DQ be the linear market demand curve. Suppose firm A enters first in the market and sells OQ₁ units at the price OP₁ on the basis of (MR = MC), thereby reaping monopoly profit given by the area OQ₁CP₁.

Let us now consider firm B's entry into the market. Given that firm A produces OQ₁, CQ becomes firm B's relevant market demand curve. Therefore, the best B can do acting on the basis of (MR = MC) is to market Q₁Q₂. As a result, price falls to OP₂ and the total profit accruing to both is given by the area OQ FP .   

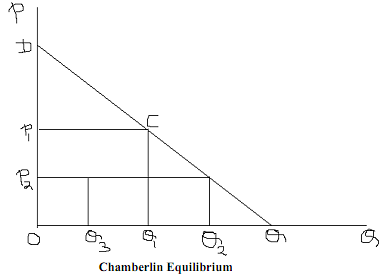
According to Chamberlin, firm A will survey the market situation after B's entry and will figure out that sharing the profit level OQ₁CP₁  is the best for either of them. Therefore, firm A would reduce it's output level from OQ₁  to OQ₃  and firm B would stick to the output level Q₁Q₂  = Q₃Q₁.  With this arrangement, the firms together produce OQ₁ and the price level is retained at OP1. Thus, we see that firm A produces OQ₃ = ½ OQ₁ and B Q₃Q₁ = ½ OQ₁. 

The total output is OQ₁  to be sold at a price OP₁ with firms A and B sharing the monopoly profit equally. Firms, in this kind of an agreement, produce more than in the Cournot case, where each one produces one-third of the total market demand.