Selling costs:

Under monopolistic competition, each firm incurs a certain amount of selling cost to inform the customer about its product. A firm under monopolistic competition has, therefore, three variables at its disposal, viz., price, quantity and selling expenditure. Let us examine how selling cost enters into the determination of equilibrium output of a firm and how the optimum amount of selling cost is determined  Under monopolistic competition, quantity demanded will be a function of both price and selling cost 

i.e.,  X = X (S, P) where  

S:  selling cost 

P: price 

X:  output demanded  

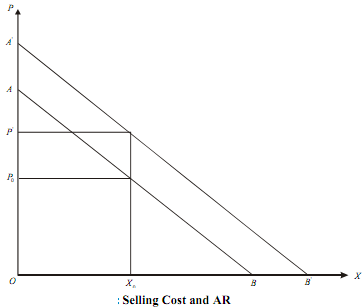
Let us assume S is fixed at level So. Then we can get different combinations of P and X for this level of S = So which satisfies the demand equation. Locus of all such points is the AR (average revenue) curve. Thus, for each level of selling cost, we will get an AR curve. This is shown in the following figure. 

AB´: AR curve when S=S_o 

A´B´: AR curve when S=S₁ 

The figure shows that for selling the same quantity (X_o), the firm can charge higher price (P_o to P₁) if it incurs higher selling costs (So to S₁). The selling cost is a function of price and quantity. Symbiotically, S = S(X, P) Therefore, Profit (π) of a firm is  

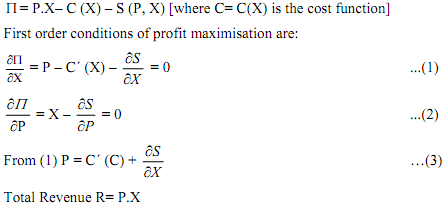
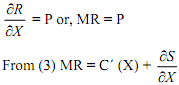
Thus, for profit maximisation marginal revenue with respect to output must be equal to marginal production cost plus the marginal selling cost. 

From X = ∂S/∂P

X may be called MR with respect to price. Thus, MR of a firm with respect to price must be equal to MSC (marginal selling cost). The following diagram explains the equilibrium of a firm 

D₀ D₀: Demand curve for selling cost S = S₀ 

D₁D₁: Demand curve for selling cost S = S₁ 

D₂D₂: Demand curve for selling cost S = S₂ 

MR₀, MR₁and MR₂ are the marginal revenues for selling cost S₀, S₁ and S₂ respectively. MC is the marginal cost curve. The second order condition required that MR increases less rapidly than MC both with respect to output and price  If the firm behaves like a monopolist, its profit maximizing output-price combination is (X_o, P_o), (X₁, P₁), (X₂, P₂) for selling cost S_o, S₁ and S₂, respectively (these are obtained by equating MR_o, MR₁ and MR₂ with MC).  

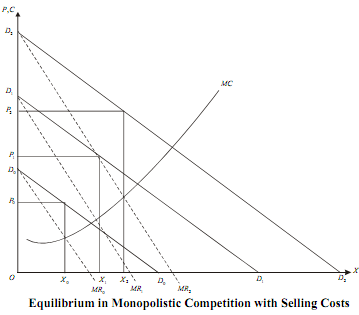
For each of these combinations, we can determine the profit. Let these be π_o, π₁, π₂, respectively for S= S_o, S₁ and S₂  

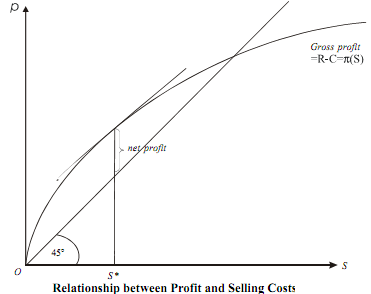
Thus, we can find a functional relationship between profit and selling costs, viz., π = π (S) 

If we plot the profit on vertical axis and selling costs on horizontal axis, the curve will look like the curve given in Figure. Clearly, where the vertical difference between π (s) and the 450 line π (S) =S is maximum,  

i.e.,  π (S) - S  

or, R - C (X) - S(X) 

or, P.X - C (X) - S(X) 

gives the optimum level of selling cost. 

Thus, the optimum selling cost (S*) is obtained when the slope of  π (S) is equal to unity.