Equation for drain current:

Let us write down the equation for drain current

I_d  =  (μ_s C_ox  W )/L  (V_gs  - V_T ) V_ds  -  ( V_ds )² /2)

This equation is called the Sah Equation after C.T. Sah, who primary described the MOS transistor operation this way back in the year 1964. It is very significant because it describes the basic behaviour of the MOS transistor. it is called the linear regime because we have a straight-line relationship between the drain current and the drain-source voltage. As V_ds starts to get larger though, the squared term shall begin to be prominent and the plot shall start to curve over as illustrated in Figure (a). Clearly, something is causing the current to drop off as V_ds gets larger. It is because the voltage difference between the gate and the channel is becoming less that means there is less charge in channel to provide conduction. We may graphically illustrate this by making the channel layer look thinner as we move through the source to the drain. Let us take a look in more detail at the place where Id becomes a maximum. We may define V_dsat as the source-drain voltage where I_d becomes maximum. We can find this by taking the derivative of Id with respect to V_ds and setting the derivative to 0.

        d/ dV_ds(I_d­) =0       = (μ _S C_ox   W  /L ) (V_gs - V_T - V_dsat)

On dropping constants :

 V_dsat  = V_gs  - V_T

Rearranging this equation provides us a little more insight into what is going on.

V_gs  - V_dsat  = V_T = V_gc  (L)

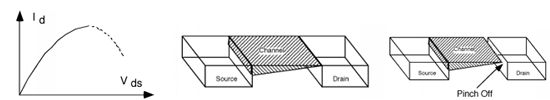
(a)                                                       (b)                                     (c)

Figure: (a) I-V Characteristics Showing Turn-over; (b) Effect of Vds on the Channel; and (c) Channel in Pinch-off