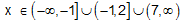Wavy curve method:

In order to compute the inequalities of the form

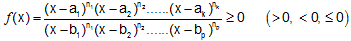
where n₁, n₂, ....... , n _k , m₁, m₂, ....... , m_p are natural and real numbers and a₁, a₂, ... , a_k, b₁, b₂, ..., b_p are any real number such that a_i ≠ b_j where i = 1, 2, 3, ....k and j = 1, 2, 3, ....p.

Method:

Step - 1-> First place all values of x at which either denominator or numerator is becomes zero, that denotes a₁, a₂,....., a_k, b₁, b₂, ....b_p in ascending order say c₁, c₂, c₃,....... c_{p + k}. draw them on real line

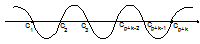
Step -2 -> Value of x number at which numerator tends to zero could be remarked with dark circles.

Step - 3 -> All pints of discontinuities (x at which denominator tends to zero) could be remarked on number line with empty circles. Calculate the value of ¦(x) for any real number bigger than the right most checked number on the number line.

Step - 4 -> From right to left presented a wavy curve (beginnings above the number line in type of value of ¦(x) is positive in step-3 otherwise from below the number line), going thoroughly all the checked points. So that when goes through a point (exponent whose related factor is odd) intersects the number line, and when going thoroughly a point (exponent whose related factor is even) the curve doesn't cut the real line and stay on the similar side of real line.

Step - 5 -> The suitable intervals are selected in accordance with the sign of inequality (the function ¦(x) is positive wherever the curve is over the number line, it is negative if the curve is searched below the number line). Their union shows the solution of inequality

Problem: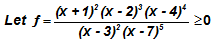       

Solution:            

Step - 1 -> Create on real line all x at which numerator tends to zero with dark circles.

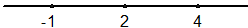
Step - 2-> present point of discontinuity (value of x at which denominator becomes zero) with empty circles

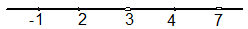
Step - 3 -> Calculate ¦(x) for x > 7, ¦(8) > 0

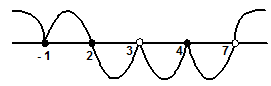
Exponents of given factors of -1, 3, 4 is even, therefore wave can not modify the direction at those points.

Therefore