Acceleration Is A Vector:

Acceleration is a vector quantity. At times the magnitude of the acceleration vector is known as acceleration, and is denoted by lowercase italic letters like a. Technically, though, the vector expression must be used; it is denoted by lowercase boldface letters like a. In the illustration of a car driving all along a highway, assume that the speed is constant at 25 m/s which is as shown in figure below. The velocity changes whenever the car goes around curves and also when the car crests a hilltop or bottoms out in a ravine or valley (though these cannot be shown in this 2-dimensional drawing). When the car is going along a straightaway and its speed is increasing, then the acceleration vector points in similar direction which the car is traveling. Whenever the car puts on the brakes, still moving all along a straight path, then the acceleration vector points precisely opposite the direction of the car's motion. Acceleration vectors can be demonstrated graphically as arrows. In figure shown below, three acceleration vectors are shown around for a car traveling along a curving road at a constant speed of 25 m/s. Three points are shown, known X, Y, & Z. The corresponding acceleration vectors are x, y, & z. Acceleration only occurs where the car is following a bend in the road. At point Y, the road is necessarily straight, therefore the acceleration is zero. This is as shown as a point at the origin of a vector graph.

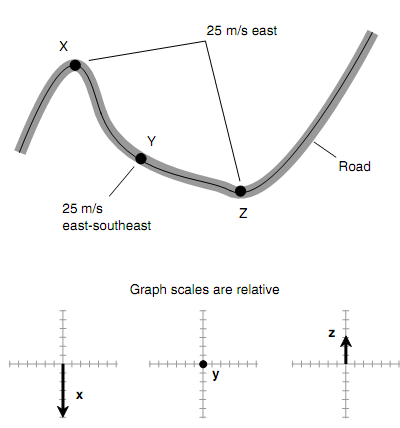
Figure: Acceleration vectors x, y, & z for a car at three points (X, Y, & Z) along a road. Note that y is the zero vector as there is no acceleration at point Y.