Goodness of Fit Tests

Except for the final step in the above approach, all other steps are familiar to us. Hence the final step in the selection of a theoretical distribution, i.e. to perform a statistical test for goodness of fit is explained here. Such a test compares a null hypothesis (H₀) with an alternative hypothesis (H₁ or H_a) having the following form:

H₀: The failure times came from the specified distribution.

H₁ or H_a: The failure times did not come from the specified distribution.

The test consists of computing a statistic over a sample of failure times. This statistic is then compared with a critical value obtained from relevant statistical tables. Generally, if the test statistic is less than the critical value (table value), the null hypothesis H₀ is accepted; otherwise, the alternative hypothesis H₁ is accepted.

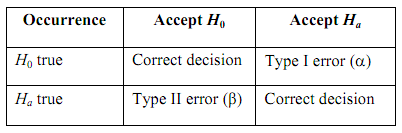
The critical value depends on the level of significance of the test and the sample size. The level of significance is the probability of erroneously rejecting the null hypothesis in favour of the alternative hypothesis. Table shows the four possible cases that may occur. Because of the randomness inherent in the sampling process, the test statistic has a probability of exceeding the critical value even though H₀ is true. This results in a Type I error having a probability of occurring equal to the level of significance (α). It is also possible for the test statistic to be less than the critical value even though H₁ is true. This results in a Type II error. It occurs with a probability that is usually controlled indirectly by the specification of the level of significance and the sample size.

                                                         Table: Decition Table for Hypothesis Tests

There are two types of goodness of tests available:

• General tests, and
• Specific tests.

A general test is applicable to fitting more than one theoretical distribution, and a specific test is tailored to a single distribution. If available, specific tests will be more powerful (have a higher probability of correctly accepting/rejecting a distribution) than general tests. What follows is a discussion of a general test, the Chi-Square goodness of fit test, and particular tests for the Exponential, Normal and Lognormal, Weibull.