Different mobilities:

The answer lies in the fact that different ions have different mobilities in solution. The mobility of an ion in solution is mainly dependent upon the size of the hydrated ion. The ionic mobility is defined as the velocity with which an ion would move under a potential gradient of 1 V m^-1 in a solution. It gives a link among theoretical and measurable quantities. For example, ionic mobility, (u), is associated to limiting molar ionic conductivity (Λ ∞) by the following equations:

Λ₊^∞ =  z₊u₊F and Λ^∞ ₊     = z_-u_-F

where z₊ and z_- are the valency of the ions, u₊ and u_- represent the ionic mobilities and F is the Faraday constant. In the given equation, if one of the two quantities, Λ ∞ or u, is known, the other can be calculated.

To find the values of Λ₊^∞ or Λ₊^∞, we define yet another quantity, called transport or transference number of an ion indicated by the symbol t₊ or t_-. It is defined as the fraction of the total current carried by an ionic species and can be expressed mathematically as,

t₊= Λ₊^∞ /Λm  and     t_ = Λ_-^∞ /Λm

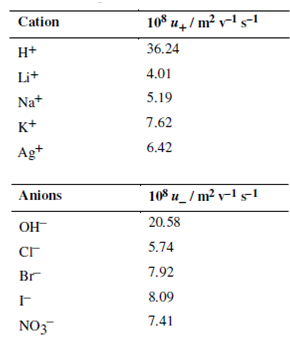
The transport number and the limiting molar conductivity are measurable quantities. Therefore, the molar ionic conductivity value can be calculated from Eq. (4.14). The limiting molar conductivities of some common ions are given in Table 4.2. These values are important in predicting the molar conductivity of electrolytes and course of conductometric titrations. At last, once the molar ionic conductivity value is obtained, we can then make use of Eq. to calculate the ionic mobility. Some typical values of ionic mobility (in infinite dilute solutions) are listed in Table.

Table:  Limiting ionic mobilities in water at 298 K