Rotation of Rigid Bodies:
A rigid body can rotate about an axis (i) passing through the mass centre (ii) not passing through the mass centre of the body. In general, the common problems occurring in engineering are of the category (i), i.e. rotation about an axis passing through the mass centre.
Consider a body as shown in Figure. The external forces acting on the body are such that they constitute a couple of magnitude M and rotate the body about an axis passing through O which is also the mass centre of the body.
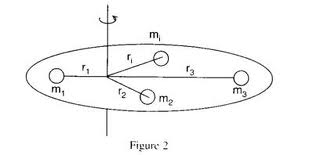
A particle of mass dm is situated at k. This particle shall traverse a circle of radius r when it rotates about an axis through O.
Figure shows the plan of the path traversed by k which shall be a circle.
As the particle k rotates about O with ω as angular velocity and α as angular acceleration, two components of acceleration (i) normal component = r ω2 and (ii) tangential component = r α will be acting on this particle which are shown in Figure.
The inertia forces dm r ω2 and dmr α are illustrated in Figure. These inertia forces shall be in the opposite direction of acceleration acting on the body.