Q. Supposing the diodes to be ideal, discover the values of I & V₀ in the given circuits.

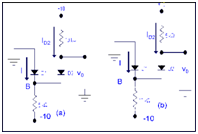
 Solution :

            For the given circuit in the diagram (a) we shall suppose that both of diodes are conducting. It follows that V_B = 0 & V₀ = 0. The current through D₂ can now be finding from

                                                             I_D2 = (10 - 0)/10

                                                                    = 1mA

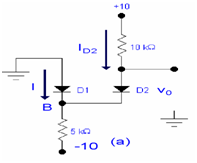
                        Writing down node equation of B,

                                                  I + 1 = 0 - (-10)/5

Results in I =1mA. Therefore D₁ is conducting as originally supposed, and the ultimate result is

                                                            I = 1mA

                                                                      &                 

                                                                        V=0

For the circuit in diagram (b), if we suppose that both of diodes are conducting, then V_B =0 and V₀ = 0 .  The current in D₂ is got from

                                                 I_D2 = (10 -0)/5 = 2mA

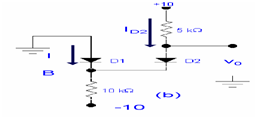
The node equation of B is

                                                 I + 2 m = 0 - (-10)/10k

            That yields I = -1mA. As it is not possible, our assumption is not right.

             We begin again, supposing that D₁ is off & D₂ is on.

            The current I_D2 is indicated by

                                                 I_D2 = 10 - (-10)/15= 1.33mA

            & the voltage at node B will be

                                                V_B = -10 + 10 x 1.33 = 3.3 V

            Therefore D₁ is reversed biased as supposed, and the last result is I =0 & V₀ =3.3 volts