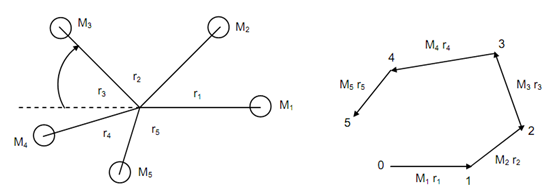
Several Masses Revolving In Same Transverse Plane:

A number of masses contain weight M₁, M₂, etc. may be associated to the shaft in the similar transverse plane such that their respective centres of gravity are at distances of r₁, r₂, etc. Each masses can be placed at its own angular location in the transverse or radial planes like depicted in Figure 4(a). The unbalance because of each mass shall be proportional to the product of mass and radius (that means. M r). If we desire to ascertain the net effect, it shall be an unbalanced force, and then we draw a polygon that does not close. The resultant unbalanced is equivalent to the closing side of the polygon exists. In this case the closing side is 5 - 0. Therefore a force equivalent to 5 - 0 in the direction 5 to 0 that close the polygon. So balancing mass might be associated parallel to 5 - 0 and the length of this side shall be the product of mass and radius.