Vectors in the RL Plane:

Engineers like to represent points in the RL as vectors. This gives each point a definite magnitude and a precise direction.

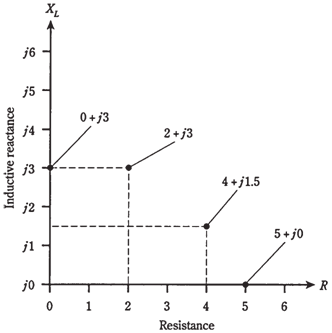
Figure   Four points in the RL impedance plane. See text for discussion.

In Figure given above, there are 4 different points shown. Each one can be represented by a certain distance to right of the origin (0,0), and a specific distance upwards from origin. The 1st of these is the resistance, R, and the 2nd is the inductive reactance, XL.  Therefore, the RL combination  is a 2-dimensional  quantity. There is no way to uniquely define RL combinations as the single numbers, or scalars, as there are 2 different quantities which can vary independently.

Another way to think of these points is to draw the lines from origin out to them. Then you can think of points as rays, each having a some specific length, or magnitude, and a definite direction, or the angle counterclockwise from resistance axis. These rays, going out to points, are vectors. You have already been introduced to these things.

Vectors seem to engender the apprehension in some people, as if they were invented by the scientists for perverse pleasure of befuddling ordinary folks. This attitude is entirely groundless. Just think of the vectors as arrows which have a certain length, and that point in some direction.

The points of Figure are shown as vectors. The only difference is that there is some more ink on paper.

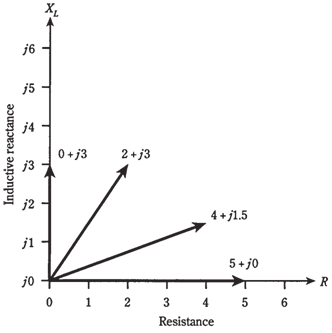
Figure--Four vectors in RL impedance plane.