Time Constant:
The value of the time constant is directly proportional to the inductance and inversely proportional to the resistance. The time constant can be found using Equation (3-5) if these two values are known.
TL = L/R (3-5)
where
TL = time constant (seconds)
L = inductance (henries)
R = resistance (ohms)
A voltage drop across an inductor is directly proportional to the product of the inductance and the time rate of modification of current by the inductor, as display in Equation (3-6).
VL = L (? I/?t) (3-6)
where
VL = voltage drop across the inductor (volts)
L = inductance (henries)
(?I/?t) = time rate of change of current (amp/sec)
After five time constants, circuit parameters generally reach their last value. Circuits which hold both resistors and inductors are known as RL circuits. The subsequent example will demonstrate how an RL circuit reacts to modify in the circuit that was show in the Figure.
1. Initially, the switch is in Position 1, and no current flows by the inductor.
2. Whenever we move the switch to Position 2, a battery attempts to force a current of 10v/100 = 0.1A by the inductor. Other than as current starts to flow, the inductor produced a magnetic field. As the field raise a counter EMF is induced which opposes the battery voltage. Like a steady state is reached, a counter EMF goes to zero exponentially.
3. Whenever the switch is returned to Position 1 in the magnetic field collapses, inducing an EMF which tends to manage current flow in the similar direction by the inductor. Its polarity will be opposite to which induced when the switch was placed in Position 2.
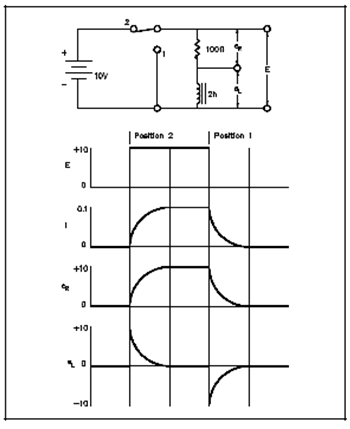
Figure: Voltage Applied to an Inductor