Connection in Three-Phase System:

The three elements that means three phases of 3-phase source or three impedances of 3-phase load may be inter-connected in two different ways called

1. Star connection, and

2. Delta connection.

In star connection similar ends the 3-phases are joined together to compose the neutral as shown in Figure (a), star connection is mention by Y and the common or neutral point is denoted by n, it is also called star point.

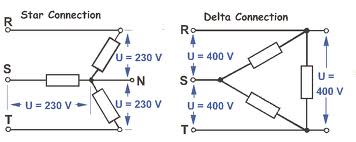
 (a) Star Connection    (b) Delta Connection

In delta connection three-phases are connected in series, end-to-end to compose a closed mesh as illustrated in Figure (b). Two dissimilar ends are joined together. Delta connection is mentioned by Δ.

It may be shown that for 3-phase balanced system, the sum of instantaneous values of currents or voltages is always zero. Let instantaneous 3-phase voltages of a balance system are represented by :

V_a  = V_m  sin ωt , V_b  = V_m  sin (ω - 120^o ) , V_c  = V_m  sin (ω - 240^o )

then, at any instant,

v_a  + v_b  + v_c  = V_m  sin ωt - V_m  sin (ωt - 120^o ) + V_m  sin (ωt - 240^o )

= V_m [sin ωt + sin (ωt - 120^o) + sin (ωt - 240^o)]

= V_m [sin ωt + 2 sin (ωt - 180^o) cos 60^o ]

= V_m [sin ωt - 2 sin ωt cos 60^o]

= 0

Likewise, for 3-phase currents of a balance system, we can show that

i_{a +} i_b  + i_c = 0

It is the important property of 3-phase balanced system which has made possible the star and delta connection of three phases, without any circulating currents.