Step 2:

The equation that gives the optimum speed for minimum cost per component is given by

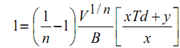            ------------------ (12)

To know optimum V, 1/n and B should be known. These two unknowns can be determined from the following relationship.

T =       B/ V _{1/ n}                   ------------- (8)

 where, T =  tool life in min, and V = velocity in m/min.

Let V₁ be the cutting speed at N₁ and V₂ be the cutting speed at N₂. Then,

V₁ = πDN₁ = π × 76.2 × 10^-3 × 330 or, V₁ = 78.998 m/min.

V₂ = πDN2 = π × 76.2 × 10^-3 × 535

or, V₂ = 128.07 m/min.

T_c1 ≈ T_ac

=          l/ f _N1

=          0.1524/3.81×10^{- 4} ×330

T_c1 = 1.21 min

Therefore, T₁ = time per component x total no. of components

= 1.21 × 620

or,        T₁    = 750 min

T_c2 = l/ f _{N 2}

=          0.1524 / 3.81×10^{- 4} ×535

T_c2 = 0.748 min

T₂ = 15 × 0.748

or, T₂ = 11.22 min