Current flow

If a conducting or semiconducting path is given between 2 poles having a potential difference, charge carriers will flow in the attempt to equalize the charge between the poles. This flow of electric current will continue till the path is given, and as long as there is a charge difference present between the poles.

At times the charge difference is equalized after a while. This is the case, for instance, when you touch a radiator after shuffling around on carpet in your hard-soled shoes. It is true in a lightning stroke. In these instances, the charge is equalized in the fraction of a second.

The charge can take longer to be used up. This will happen if we short-circuit a dry cell. Within few minutes, or maybe an hour, the cell will "run out of juice" if you put a wire between positive and negative terminals. If you put a bulb across the cell, say with a flashlight, it takes an hour or 2 for the charge difference to drop to zero.

In household electric circuits, the charge difference will never equalize, unless there's a power failure. Certainly, if you short-circuit an outlet, the fuse or breaker will blow or trip, and charge difference will drop to zero immediately. But if you put a 100-watt bulb at outlet, the charge difference will be maintained as current flows. The power plant can keep a potential difference across light bulbs indefinitely.

You may have heard that "It's current, not the voltage, kills," concerning the danger in the electric circuit. This is a truth, but it plays on semantics. It is like saying "It is the heat, not fire, which burns you." But there can be a deadly current only if there is enough voltage to drive it through the body. You do not have to worry while handling the flashlight cells, but you would better be careful around household utility circuits. A voltage of 1.2 to 1.7 V cannot normally pump a dangerous current through you, but a voltage of 117 V always can.

Through an electric circuit having constant conductivity, the current is proportional to applied voltage. Which means that, as you double the voltage, you double the current; as the voltage is cut in half, the current is cut in half. The given Figure shows this relationship as a graph in general. But it supposes that the power supply can provide the required number of charge carriers. This rule holds within the reasonable limits.

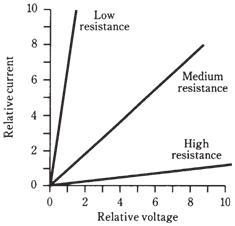
Figure: Relative current versus relative voltage for different resistances.

When you are charged with the static electricity, there are not many charge carriers. A dry cell runs short of energy after some time, and can no longer deliver as much current. All the power supplies have their limits in terms of the current they provide. A power plant, or a power supply which works off of the utility mains, or a large electrochemical battery, has a huge capacity. You can say that if you cut the resistance by a factor of 100, you will get 100 times as much current. Or perhaps even 1000 or 10,000 times the current, if resistance is cut to 0.001 or 0.0001 its former value.