Principal - agent framework:

We start with  the  situations  of  asymmetric information in which one  agent knows something that another does not prior to contracting. One person, the principal, wants to delegate a task to another,  the  agent, to undertake some action, which is costly to her (the agent). See that principal's welfare depends on what the agent does.

What we observe in the principal-agent problems is there is  a contract between the principal and the agent; information gap exits between the principal and the agent; and such a gap has implications for the decision of the contract they sign. Note that a contract  is an agreement between  the two parties  to  act  in some specified way. The agent is willing  to undertake the  task as long as her  net utility from performing it is at least as large as she can earn frorn her next best opportunity. The agent, if hired, has  to decide whether to work hard  o:  not. Hard work involves incurring disutility for the agent. So all other things equal, she would prefer not to work hard. As a result, the value of the work would be lower  and the principal will get very  little from  the deal.  In  order to ensure that  the contract results in gainful trade, the principal must make provisions, may be by providing incentives (like bonus), so that the agent puts in her best efforts.

Implicit in signing of a contract is the fact that it is enforceable. Without such a provision no one will enter a contract. Courts are entrusted with the task of enforcing it. To avoid its no-enforceability or even to minimise the chance of court cases, which are expensive  and  time consuming, attempts are made  to design contract  in  such a way  that each party  chooses to adhere to  its terms and conditions. In order words, contracts are designed  to be self-enforcing. Once  we  limit ourselves to a self-enforcing contract,  the  principal-agent framework reduces to one of principal offering the agent a contract, which is either accepted or  rejected by the agent. 

Let  us  present  the  principal-agent problem  in  terms  of  a  manager-worker example: the manager wants the worker  to put  in as much effort as possible for  producing maximum output.  However,  the  worker rationally  wants  to make  a choice that  would  maximise her  own utility given the. effort and incentive  payment  scheme. We  use  the notation of  Varian  (1992) while
introducing  the problem.

Let  x be  the output received by  the principal. The agent has a set of feasible actions A  from which she chooses actions  a  and  b .  If we assume that is no uncertainty, output will be completely determined by  the actions of the agent. We write output-effort relationship as x = x(a),  lost of action  a  as c(a) and incentive payment from the principal to the agent as s(x). In  this formulation, the  utility  function of the principsl is  given  by  output minus the incentive payment,  i.e., x  -  s(x). The utility function of the agent, on the other hand, is the decided by  the  difference between  incentive payment and the cost of the action, [s(x) -  c(a)] giving her utility function: u[s(x) -  (x)].

The maximisation problem of the principal is given by

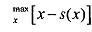
Subject  to  the constraint  s(x)  - (c(a)),  imposed by the agent's optimising behaviour

There are two types of constraints when the agent is involved. First, the agent may  have another opportunity available  to her, which  gives  her  some reservation level of utility. In order that  the  agent is willing to participate in the contract, the principal must be willing to give her at least this reservation level.  This  is called  the  participation constraint  or individual  rationality constraint. Secondly, given the incentive schedule chosen by  the principal, the agent will  pick  the  best  action  for  herself. This  type of  constraint is  called incentive  compatibility constraint.  The  second  constraint implies that irrespective  of  the  terms  of the  contract, the agent will maximise her own utility.