Load Acting Eccentric to One Axis:

The bending couple P × e will cause longitudinal tensile & compressive stresses. The fibre stress because of bending f₀, at any distance 'y' through the neutral axis is given by,

f_b  = ( M/ I _xx) × y = (P × e × y )/ I _xx  (tensile or compressive)    ------ (1)

Therefore, the total stress at any section is given by following

f  =  f₀ ±  f   = (P/A) ± ((P × e × y )/ I xx)  --------- (2)

f  = (P/A) ±  (M/ Z _xx) [where P × e = M and   I _xx /y = Z _xx  (the section modulus)].

The extreme fibre stresses are given by following,

f _max      =  f₀ +  f b  = P /A +  (M/ Z _xx)

f _min      =  f₀ - f b  = P /A -  (M/ Z _xx)  ----------- (3)

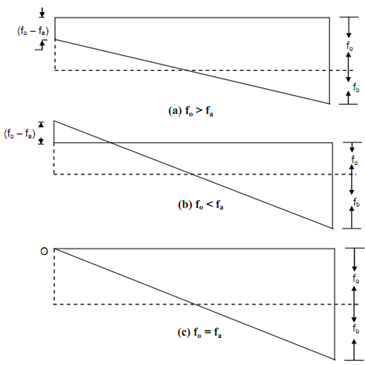
If f₀ is greater than f_b, the stress during the section shall be of the similar sign. If though, f₀ is less than f_b, the stress shall change sign, being partly tensile & partly compressive across the section. Therefore, there might be three possible stress distributions as illustrated in Figures (a), (b) & (c). You might observe from Figure (c), that while

f₀ = f_b, f_max = 2f₀ and f_min = 0.

      Figure