Classical Theory of Bending:

You are already acquainted with the theory of simple bending of beams, where the bending stress 'σ' at a distance y from the neutral axis of the section is given by the equation:

σ/y = M/I

Also the shear stress, τ, is given by

τ = F . A y¯  /I . by

Whereby is a width of section at that distance z from neutral axis (NA) and  y¯  is centroidal distance of hatched area from neutral axis.

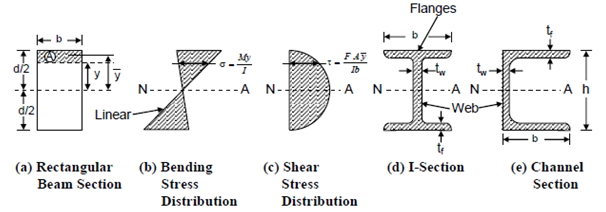
To a rectangular beam the stress distribution is shown in Figures (a) to (c).

As the bending stresses near the neutral axis are very small (Figure 1(b)) the contribution of the material there to resist external bending moment is small. Hence, the beam and joists sections are generally of thin web type I or channel sections (Figures (d) and (e)) where the wider flanges carry most of the bending resistance. Instead, the shear stress is maximum at the neutral axis (Figure (c)) hence it is the web, that takes most of the shear stress and contribution of flanges is very small.

Figure