Cantilever Beam with a UDL on Some Portion:

Udl on the right half portion id following:

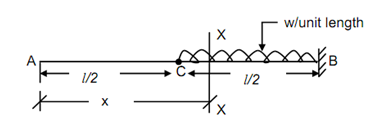
Consider a cantilever beam loaded with a Udl of w/unit length on portion CB as illustrated in Figure

Consider a section X-X at a distance x from left end.

Moment,

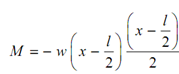
=-(w/2)(x-(l/2))²          (Hogging BM)

Figure

The governing equation for deflection is following:

EI (d ² y/dx²)     = M = -w/2[x-(l/2)]²

Integrating the Eq. (129), we can get

EI (dy/ dx)        =- w/ 6[ x- (l/2)]³ + C₁

EIy (dy/dx) = -w/24 [  x -(l/2)]⁴+ C₁x+C₂

The constants C₁ and C₂ can be found from boundary conditions. The boundary conditions are :

 At B,  x = l, dy / dx = 0                   -------------(1)

At B, x = l, y = 0                      --------- (2)

Applying the boundary condition (1) into the Eq. (9.130), we can get

0 = -w/ 6[l-(l/2)]³    + C₁

∴          C1 = wl³ / 48

Applying boundary condition (2) into the Eq. (9.131), we can get

0= -(w/24)(l-(l/2))⁴+(wl³/48 )´l + C₂

=(wl⁴/(24´16 ))[-1+8]+C₂

C₂=-7wl⁴/384

The slope and deflection equations shall be :

 EI( dy/dx)        =- (w/6)[x-(l/2)]3 + w l³ /48

EIy =-  (w/24)[x-(l/2)]⁴+wl³/48x-7wl⁴/384

 Slope at (x = 0),

θ_A = + 48 wl⁴ /48EI

Slope at C (x = l/2)  ,

  θ_c = + 48 wl⁴ /48EI

Deflection at A (x = 0),

y _A  =- (7  / 384)  (wl ⁴/ EI)

Deflection at C (x = l/2)  ,

EIy_c  =+ (wl3/  48)× (l /2) - (7wl ⁴/384)

=  (wl ⁴ /384)(4 - 7) =- 3 w l ⁴/384

∴   y _C        =- (3/384)(wl⁴/  EI)