Consumption Function:

Consumption function  shows the  relationship  between c  and  y. Our consumption function should depict  the above  features.  If we ignore  taxes and subsidies  by the government, then consumption function  in its simplest  form  is  given by

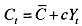
where C_t is  current consumption and Y_t is  current  income. The consumption  function given in equaction is a straight line with  intercept C' and slope  c.  Here C'  is  the  threshold or minimum consumption when income  is  zero. Since  a  household spends  only apart  oKits income on consumption the value of c should be less  than 1. Moreover, c cannot be negative. Thus  c  remains betwen 0 and 1,  that is, 0 <  c <  1  . We observe  that c  is  the  MPC since  c = ΔC / ΔY  .Notice an  important feature  of  the consumption function,  that is the average propensity to consume  (C_t /Y_t)  falls  as income rises.  

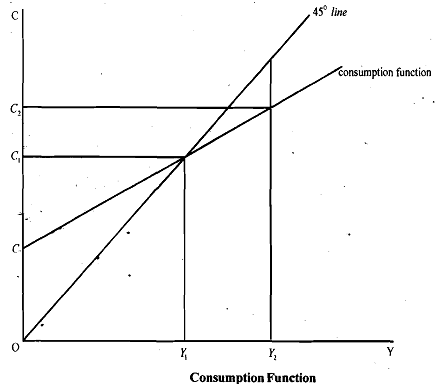
In Fig. we depict the consumption function. Here we draw a 45⁰  line along which whatever is earned  is  consumed,  that is, C =  Y When income  level  is Y₁, consumption  equals  income as  the consumption hction  intersects  the 45⁰ line. this is level of  income  is  called  'breakeven income', and on  the avexage, a  family  having income Y, consumes whatever Y₁ earns. When income  is  less  than Y₁ consumption  level of  the household is more than  its income. On  the other  hand, when income  is more than  Y₂ consumption  is less than income. For example, in Fig.  when income is Y₂ consumption  is C₂. Notice that when  income exceeds Y₁  we have C <  y .