Strain Energy in Bending:

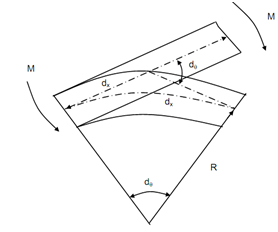
Let the element now be subjected to a constant bending moment M causing it to bend into an arc of radius R and subtending an angle dθ at the centre, as in Figure 8.4. The beam shall also have moved through an angle dθ.

Strain energy of the element, dU = work done

= (½) × moment × angle of rotation

Figure

= (1/2) M ×dq

= ( ½) M × ds (since, ds = R d θ)

= (½) M × (M /EI) × ds (since, M /I = E/R)

= M ² ds / 2 EI

Total strain energy in bending,

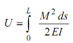
For small curvatures this reduces to

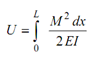
here dx is the undeformed element length.

If M is constant throughout the length L, after that

U = M ² L /2 EI