Samuelsons Criteria:

A way out of Gorman's  intransitivity problem  has  been proposed  by  Paul Samuelson,  popularly known  as  the  Samuelson  Criteria.  The  Samuelson criteria argues that a situation G is preferred to a situation D if all hypothetical redistributions  from  situation  G  will  achieve  utility  allocations  that are superior  to  some hypothetical  redistributions  from  situation D  and  that  no
hypothetical redistribution from  situation D will yield utility  allocations that are unattainable through any hypothetical redistributions from  situation G.  in other words,  the  utility  space  lies  everywhere above  UPF_D. In production space, it  requires that PPF_G  intersects the interiors of CIC_D  and the interior of any other CI_C tangent to PPF_D.  Clearly, this will often require that PPF_G  lies

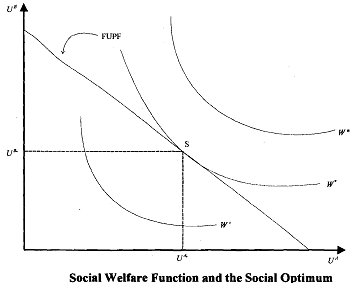
everywhere above PPF_D  - as in Figure . Naturally, if we deal exclusively with PPF_s of this type, neither Scitovsky reversals nor Gorman intransitivities arise. But  it also obviously highly restrictive as  it  rules out quite reasonable  situations . Thus, in  this sense, the  Samuelson  criterion  is  far more restrictive  than  the  Kaldor,  Hicks  or Scitovsky double criteria.