Kaldor-Hicks Criteria:

Let us consider the Edgeworth-Bowley box as shown in Figure below. It is evident that allocations D and F (and  indeed, all  allocations  in  the "lens" formed by u^A(E)  and u^B(E))  are Pareto-superior  to E, but allocation C cannot be compared to either D, F or E.

An  alternative criterion of  judging whether an  allocation was "preferable"  to another was proposed by Nicholas Kaldor in his article, 'Welfare Propositions of  Economics and Interpersonal  Comparison of  Utility', published  in  The Economic Journal, September, 1939. According to Kaldor's welfare criterion, if a certain change  in  economic organisation or policy makes some  people better off and others worse off, then that change will increase social welfare, if those who gain  from the reorganisation could compensate the losers and still be better off than before.  In other words, Kaldor argued  that an allocation is preferred  to another  if by  moving  from  the  second  to the first, the  "gainer" from  the move can, by  a  lump-sum  transfer, compensate the "loser"  for her loss of utility and still make a gain in utility for herself. Hicks stated that, 'ifA is made so much better by the change that he  could compensate B  for  his loss and  still  have  something  left  over,  then  the  reorganisation  is  unequivocal improvement'.  To put it in other way, a change is an improvement  if the losers in the changed situation cannot profitably bribe the gainers not to change from the original situation. Hicks has given  his criterion from the loser's point of view, while Kaldor had  formulated this criterion  from  the gainer's  point  of  view. Thus, the  two  criteria  are  really the same though they are exposited
differently. That is why they  are generally called by  a single name,  'Kaldor- Hicks Compensation Criteria'.

Suppose we want to reorganise the allocation by moving from allocation E to allocation C.  This  shows that, individual A gains  in  utility (from u^A(E)  to u^A(c))  and individual B loses (from u^A(E)  to u^B(C)).  This implies that, E and C are not Pareto-comparable. However, if we move to allocation C, individual A can pay  individual B a portion of her gains so  as to keep individual B at her old utility level u^A(E).  For instance, A can pay B the amount X^AC - X^AF (thus she moves to point F) so that B retains the same old utility  level uB  (E) while the utility of A is now u^A(F).  As the utility of A at allocation C is greater than that at F, (X^AC - X^AF),  individual A makes a net gain X^AC > X^AF  plus whatever she gained  in terms of good Y. Thus, as  shown in  the figure when u_A(F)> u_A(E)  it  is worthwhile for her  to propose moving  to C  and then paying individual A the amount X^AC > X^AF,  thereby moving the economy  to F.

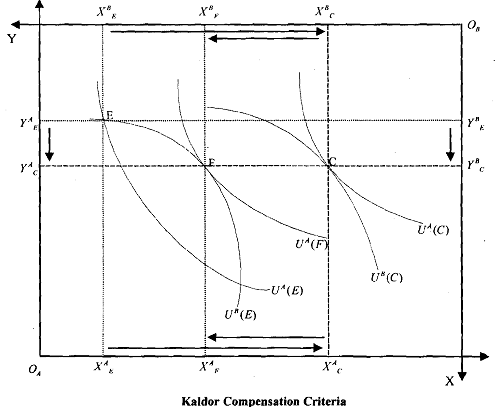
Now, if the Kaldor compensation criteria implied merely that we moved from E to F, then  it  is not an improvement on the Pareto criterion as F is obviously Pareto-superior  to  E.  However,  Kaldor's  innovation is  to  propose  that allocation C is superior to that of E because it is  possible  for A to compensate B and still be better off. The crucial point is that A can compensate B, and not that A will compensate B. Thus, the move from E to C is actual, but the move from C to F is only hypothetical. Thus, Kaldor proposed that we can compare Pareto-incomparable points via the "hypothetical  cornpernation"  test. In  sum, an allocation  is  preferable  to  another  if it is  possible  to  hypothetically redistribute goods so  that a Pareto-improvement occurs.