Second model of Chamberlin:

In this model, it is assumed that the number of firms is such that there is no supernormal profit. Therefore, there is no entry and exit.

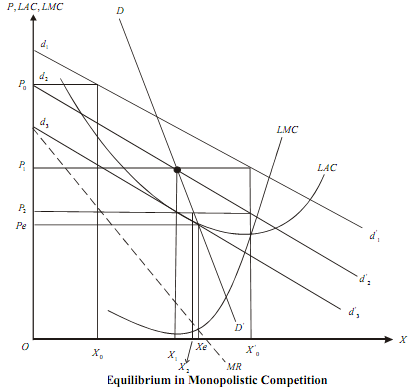
In the model we consider a demand curve labelled DD´ shown in the following Figure. The demand curve shows the actual sales of the firm at each price after accounting for the adjustments in price made by other firms. DD´ is called 'share of the market demand curve: DD´ is obtained as a locus of points of shifting dd´ curves, as competitions also simultaneously charge their price. DD´ is steeper than dd´ curve (why?). A movement along DD´ shows changes in actual sales of existing firms as all of them adjust their price simultaneously (and more importantly) identically, with their share remaining constant. Shift in DD´ is caused by entry and exit of firms.  

We start with the non-equilibrium position of a firm (X₀, P₀). The firm in an attempt to maximise profit lowers its price to P1 expecting to increase the sell. However, all the firms adjust to this price change by lowering theirs by the same proportion. As a result, d1d1  shifts downward to d2 d2 and instead of X₀¹ , only X₁ amount of output is sold (notice that: (X₁, P₁) is on DD´)

Again, in order to maximise profit, firm reduces its price once more to P_2, but as a consequence of the price adjustment by the others, it could sell only x₂ (though wished to sell X¹_o ). Here we assume that  the firm is naive and does not take lessons from the past. This process ultimately stops when dd´ has shifted enough to the left to be tangent to the LAC.