Capacitive Time Constant:

While a capacitor is linked to a DC voltage source, it charges extremely rapidly. The capacitor would become charged almost instantaneously if no resistance was present in the charging circuit. A Resistance within a circuit will cause a delay in the time for charging a capacitor.  The exact time needed to charge a capacitor depends on the R (resistance) and the C (capacitance) in the charging circuit. In the Equation (3-13) describes this relationship.

T_C  = RC                                             (3-13)

where

T_C  = capacitive time constant (sec)

R  = resistance (ohms)

C  = capacitance (farad)

The capacitive time constant is the time needed for the capacitor to charge to 63.2 % of its fully charged voltage. Within the following time constants, the capacitor will charge a further 63.2 % of the remaining voltage.  A capacitor is considered fully charged after a period of five time constants that was show in the Figure.

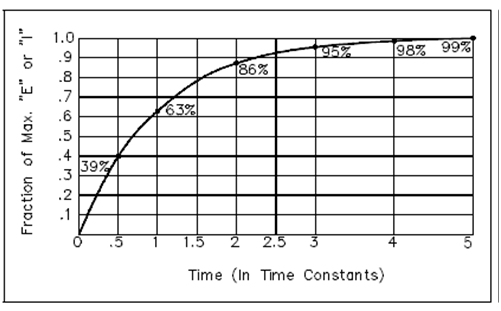
Figure Capacitive Time Constant for Charging Capacitor

The capacitive time constant also display which it needed five time constants for the voltage across a discharging capacitor to drop to its minimum value  show in the Figure.

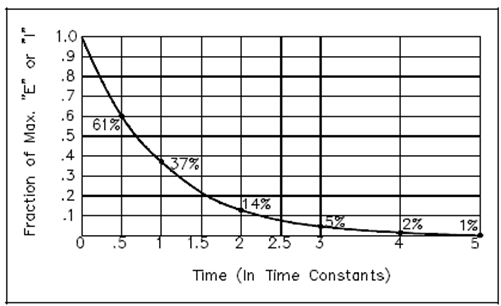
Figure Capacitive Time Constant for Discharging Capacitor