Example: Extended Bernoulli

Water is pumped from a large tank to a point 65 feet higher than the reservoir. Determine how many feet of head should be added by the pump if 8000 lbm/hr flows via a 6-inch pipe and the frictional head loss is 2 feet? And the density of the fluid is 62.4 lbm/ft³, and the cross-sectional area of a 6-inch pipe is 0.2006 ft².

Solution:

To employ the modified form of Bernoulli's equation, reference points are selected at the surface of the reservoir (i.e., point 1) and at the outlet of the pipe (i.e., point 2). The pressure at the surface of the tank is similar as the pressure at the exit of the pipe, that is, atmospheric pressure. The velocity at point 1 will be necessarily zero. By using the equation for the mass flow rate to establish the velocity at point 2:

m₂ = ρA₂v₂

Now we can employ the Extended Bernoulli equation to establish the needed pump head.

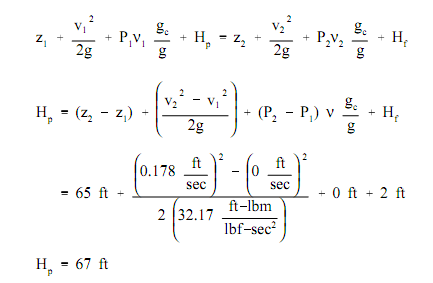
The student must note that the solution of this illustration problem has a numerical value which "makes sense" from the data given in the trouble. The total head rise of 67 ft. is due chiefly to the 65 ft. computation rise and the 2 ft. of friction head.