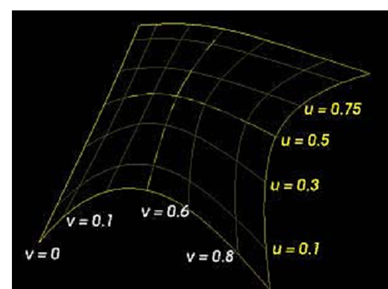
Isoparametric Curves on a Surface:

These isoparametric curves may help to render the surface. In several applications, a parametric surface is "triangulated" into triangles or polygons. After that, these triangles and polygons may be rendered extremely efficiently with existing graphic tool libraries such as PHIGS PLUS and OpenGL.

Rather than triangulating the surface, this would be easier to triangulate the domain of (u, v). We may subdivide the u-direction into m segments with u₀ = 0, u₁, . . . , u_i, . . . , u_m = 1 and subdivide the v-direction into n segments with v₀ = 0, v₁, . . . , v_j, . . . , u_n = 1. The domain is subdivided into m × n rectangles, each of which may be further subdivided into two triangles as illustrated in Figure.

Figure

Imagine ui and ui+1 are two consecutive division points in the u-direction and v_j and v_{j + 1} in the v-direction as illustrated in Figure. We contain a rectangle in the domain with vertices (u_i, v_j), (u_{i + 1,} v_j), (u_{i + 1}, v_{j + 1}) and (ui, vj + 1). We have two ways to attain the triangles because this rectangle has two diagonals. The first triangle is described by (u_i, v_j), (u_{i + 1}, v_j) and (u_i, v_{j + 1)} and the second triangle is described by (u_{i + 1}, v_j), (u_{i + 1}, v_{j + 1}) and (u_i, v_{j + 1}).