Torque:

The torque of an AC induction motor is dependent upon the strength of the interacting rotor and stator fields and the phase relationship among them. It could be calculated through using given Equation (12-3).

T = KΦI_R cosθ_R                                                          (12-3)

where

T        =           torque (lb-ft)

K         =          constant

Φ        =           stator magnetic flux

IR        =          rotor current (A)

cosθ_R   =          power factor of rotor

During normal operation, K,Φ, and cosθ_R are, for all intents and motives, constant, so in which torque is directly proportional to the rotor current.  A Rotor current increases within almost direct proportion to slip. The change within torque along with respect to slip shows that, as slip raise from zero to ~10%, the torque increases linearly. Since the load and slip are increased beyond full-load torque, the torque will reach a maximum value at about 25% slip. A maximum value of torque is known as the breakdown torque of the motor.  The motor will stall and come to a rapid stop if load is increased beyond this point. The classical induction motor breakdown torque varies from 200 to 300% of full load torque. Beginning torque is the value of torque at 100% slip

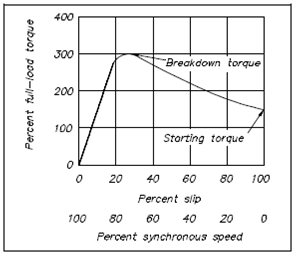
Figure: Torque vs Slip

and is generally 150 to 200% of full-load torque.  As the rotor accelerates, torque will rise to breakdown torque and after that decrease to the value needed to carry the load on the motor at a constant speed, commonly among 0-10%.