Series and Parallel Resonance:

Resonance is that condition in any AC circuit at a specific frequency, while the applied voltage and resultant current are in same phase. Thus, at resonance the power factor of the AC circuit is unity and it acts like a pure resistive circuit.

Series Resonance

In series RLC circuit at a specific frequency when inductive reactance (X_L = ωL) becomes equal to the capacitive reactance ( X _C =   1/ ω_C  ), then the circuit is said to be at resonance

                                                          X_L = X_C

 In the series RLC circuit of Figure, the circuit current I is specified by

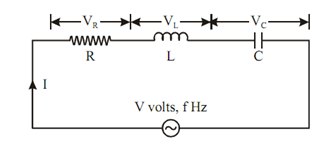
Figure: Series Resonance Circuit

I =( V/ Z)  A

where Z represents the equivalent impedance of the circuit.

Z = R + j ωL +(1/ j ωC)

= R + j ωL -(1/ ωC)j

Z = R + j ( X _L  - X _C )

where

X _L        = ωL and  X_C    =   1/ ωC

= R +  jX

where (X_L - X_C) = X (Net Reactance)

 Therefore

I =        V / (R + j ( X _L - X _C ))  = V / R +  jX    Amp

Figure: Voltage and Current Phasor

And voltage drop across resistance, R is V_R = IR (in phase with I)

 Voltage drop across inductance, L is V_L = I X_L (leading I by 90^o)

Voltage drop across capacitance, C is VC = I XC (leading I by 90^o)

The phasor diagram of the variables of the entire circuit is shown in Figure

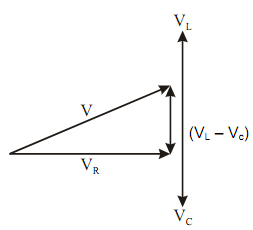
Figure: Voltage Vector Diagram of RLC Series Circuit