In a floating rate security, the coupon rate changes periodically as per the reference rate. The yield to maturity of floating rate securities cannot be calculated as the future value of the reference rates which determine the cash flows are unknown. Instead, conventional measures like spread for life, adjusted simple margin, adjusted total margin, and discount margin are used. Of these, the discount margin measure is more popular. The method for calculating discount margin is given below:

1. First, cash flows are determined assuming that the reference rate does not change over the entire life of the floating rate security.

2. Next, a margin is to be selected.

3. Then, the cash flows found in step 1 are discounted using the current value of the reference rate plus the margin selected in step 2.

4. The present value calculated in step 3 is then compared to the price plus accrued interest. If it is equal then the margin selected is the discount. If not, the process needs to be repeated with a different margin.

Let us illustrate this with an example.

Assume that the coupon reset formula for a Rs.100 par value, 4-year floating-rate security selling at Rs.98.4991 is the 6 month MIBOR plus 50 basis points. The interest rate is reset after every 6 months. The current MIBOR is 7.50%.

Table : Calculation of Discount Margin for a Floating-Rate Security

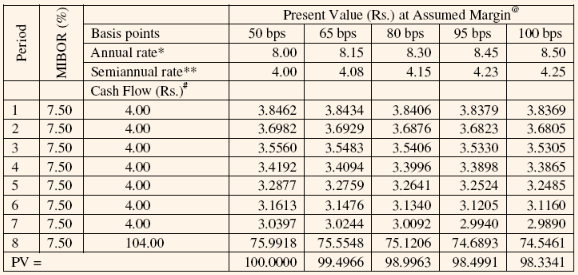
Cash flow for the period 1 to 7 is semiannual interest rate and for 8th period it is semiannual interest rate plus par value of the security.

   Annual Rate = MIBOR + Basis Points

   Semiannual rate = Annual rate / 2

   PV at assumed margin = Cash flow / (1 + Semiannual rate) ^{time period}

Discount margin as a measure of the returns form the floating rate security assumes that the reference rate will not change over the life of the security and it also does not take into consideration the cap and floor of the floating-rate securities. These are the two main drawbacks of this measure.