What is the net angle of twist at the free end:

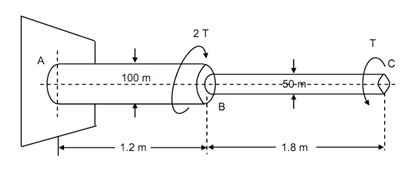
The stepped steel shaft illustrated in Figure is subjected to a torque (T) at the free end and a torque (2T) in the opposite direction at the junction of the two sizes.

What is the net angle of twist at the free end, if the maximum shear stress in shaft is limited to 70 N/mm²?

Suppose the modulus of rigidity to the 0.84 × 105 N/mm².

Solution

Torque at C = T (anticlockwise)

Torque at B = 2T (clockwise)

 Maximum shear stress, τ_m = 70 N/mm²

G = 0.84 × 10⁵ N/mm²

Assume θ be the angle of twist at C.

Free Body Diagram

A reaction torque shall be induced at section A. Consider this torque be T_A clockwise. Then total torque should vanish for equilibrium, thus,

T_A  + T_B  - T_C  = 0

∴          T_A  = T_C  - T_B  = T - 2T = - T

which means torque at A is equal to T in magnitude but opposite the supposed clockwise direction. T_A is equal to T anticlockwise as illustrated in free body diagram of Figure .

                                                      Figure: Free Body Diagram

The situation is that the equal torques T (but opposite in direction behaves on two portions). The similar magnitude of torque in BC shall induce higher stress than in AB because of diameters as illustrated in Figure. Hence, T should be determined from the portion BC.

T =  (π /16) τ_m d ³  = (π /16) × 70 × (50)³  = 17.2 × 10⁵  Nmm

J _AB=  (π /32 )× (100)4  = 982 × 10⁵  mm⁴

J _BC =  (π/32 ) × (50)⁴  = 6.14 × 10⁵  mm⁴

The angle of twist is the difference of twist of C w.r.t. B and the twist of B with respect to A. (As two are in opposite directions).

θ_BC = T L_BC / G J _BC       (anticlockwise)

θ _AC= T L_AB / GJ _AB           (clockwise)

θ=θ_AC   =   (T /G) [L_BC/ J _BC - L_AB / J _AB ]          (anticlockwise)

= (17.2 × 10⁵ /0.84 × 10⁵)[   (1.8 × 10³/ 6.14 × 10⁵ ) - (1.2 × 10³  / 98.2 × 10⁵) ]?

= 20.5 [0.293 - 0.0122] × 10^{- 2}

= 0.058 rad. = 3.3 deg.

or θ = 3^o 18′