Vectors and Matrices:

The Vectors and matrices are used to store sets of values, all of which are similar type. A vector can either be a column vector or a row vector. The matrix can be visualized as a table of values. The dimensions of a matrix are r x c, here r is the number of rows and c is the number of columns. This is pronounced as "r by c." If the vector has n elements, the row vector would have dimensions 1 x n, and a column vector would have the dimensions n x 1. The scalar (one value) has dimensions 1 x 1. And hence, vectors and scalars are actually just subsets of matrices. Here are some diagrams shown below, from left to right, a scalar, a column vector, a row vector, and the matrix:

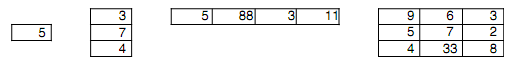
The scalar is 1 x 1, the column vector is 3 x 1 (3 rows by 1 column), the row vector is 1 x 4, and the matrix is 3 x3. All the values stored in these matrices are stored in what are termed as elements.

The MATLAB is written to work with matrices; the name MATLAB is short for "matrix laboratory." For this reason, it is very easy to generate vector and matrix variables, and there are so many operations and functions which can be used on vectors and matrices.

A vector in a MATLAB is equal to what is termed as a one-dimensional array in other languages. The matrix is equal to a two-dimensional array. Generally, even in the MATLAB, some operations which can be performed on either vectors or matrices are referred to as array operations. The word array also is often used to mean generically either a vector or a matrix.