Standard conventions in Game Theory

Consider the following table as shown below:

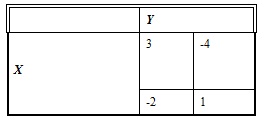
X plays row I, Y plays Column I, X wins 3 points
X plays row I, Y plays Column II, X loses 4 points
X plays row II, Y plays Column I, X loses 2 points

X plays row II, Y plays Column II, X wins 1 points

3, -4, -2, 1 are the known pay offs to X (X takes precedence over Y)

Here the game has been represented in the form of a matrix. When the games are expressed in this fashion the resulting matrix is commonly known as PAYOFF MATRIX.

Strategy: It refers to a total pattern of choices employed by any player.  Strategy could be pure or a mixed one.

In a pure strategy, Player X will play one row all of the time or player Y will also play one of his columns all the time.

In a mixed strategy, Player X will play each of his rows a certain portion of the time and player Y will play each of his columns a certain portion of the time.

Value of the Game: The value of the game refers to the average pay off per play of the game over an extended period of time.