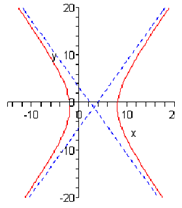
Example: Sketch the graph of hyperbolas.

                                 ( x - 3)² /25 - ( y + 1)²/49 =1

Solution

Now, notice that the y term contain the minus sign and thus we know that we're in the first column of the table above & that the hyperbola will be opening left & right.

The first thing which we must get is the center as pretty much everything else is built around that. In this case the center is (3, -1) and as always watches the signs! Once we contain the center we can get the vertices. These are (8, -1) and ( -2, -1).

Next we must get the slopes of the asymptotes. These are always square root of number under the y term divided through the square root of the number under the x term & there will always be a +ve and a -ve slope. The slopes are then ±7 /5

Now that we've obtain the center & the slopes of the asymptotes we can obtain the equations for the asymptotes. They are,

     y = -1 +(7/5) ( x - 3)      and                 y = -1 - 7 ( x - 3)

Now we can start the sketching.  We begin by sketching the asymptotes & the vertices.  Once these are done we know what the fundamental shape should look as so we sketch it in ensuring that as x gets large we move in closer & closer to the asymptotes.

Following is the sketch for this hyperbola.