Resultant of Non-coplanar Force System:

The resultant of a system of coplanar forces can be attained by adding up two forces through law of parallelogram at a time and after that combining their sums.

Analytically, the similar result can be obtained by forming the rectangular components of the forces along with any two convenient perpendicular directions. The magnitude and the direction of the consequential are found in the same way as that given for the concurrent forces, i.e.

                                tan θ_x  = ∑ F_y / ∑ F_x

The position of the line of action of R can be computed with the help of Varignon's theorem.

In particular case of non-coplanar forces, there can be parallel or non-parallel system of forces. In particular case of parallel forces, the resultant can be  (i) a single force R parallel to the system of forces, (ii) a couple in the plane of the system or in a parallel plane, or (iii) zero.

R = Σ F and it is parallel to the line of action of forces.

If          R ≠ 0 then the location of the line of action of the forces may be found out by

R × d = ∑ M o

Here,   d = perpendicular distance through the moment centre to the resultant R,

Σ M_o = algebraic addition of the moments of all of the forces w.r.t. the point under reference.

If Σ F = 0, the resultant can consist of a couple of magnitude Σ M_o.

Σ F = 0 and Σ M_o = 0, after that the resultant is zero.

For non-parallel forces, the resultant can be (a) a single force R (b) a couple, M, or (c) single force R and a couple M. In general, this is a combination of a force and a couple.

The resultant of any general force system may be attained by resolving each force into a parallel force through some of the common points and a couple. Therefore, the system is decreased to a set of concurrent forces and a set of couples. The resultant of concurrent force system may be attained by resolving the force into three mutually perpendicular axes system, x, y and z axis. Therefore, we obtain

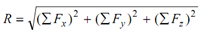
cos θ_x = ∑ F_x / R, cos θ _y =∑ Fy / R cos θ_z = ∑ Fz/ R

where ∑ F_x , ∑ F_y  and ∑ F_z are the algebraic sums of the components of all of the forces along with x, y and z axes, respectively. The angles θ_x, θ_y and θ_z are the angles that the resultant R makes respectively with x, y and z axes.