Resolving all of the forces normal to the inclined plane:

A body weighing 500 N is resting on an inclined plane making an angle of 30^o with the horizontal. The coefficient of friction is equal to 0.3. A force P is applied parallel to & up the inclined plane. Determine the least value of P while the body is just on the point of

1.      Case I : moving down, and

2.      Case II: moving up.

Solution

              The angle of friction φ = tan ^-1 (0.3)

                                             = 16^o 41′ 57′

                                                ∴ α > φ

Case I

 While the body is just on the point of moving down, the frictional force shall be acting upwards.

Resolving all the forces parallel to the inclined plane, we obtain

P₁ + F₁ - W sin 30^o = 0

∴ P₁ = 500 sin 30^o - F₁

= 250 - F₁

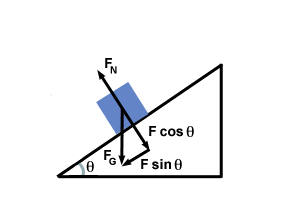
Resolving all of the forces normal to the inclined plane, we obtain

N₁  - W cos 30^o  = 0

∴ N₁  = 500 cos 30^o  = 433 N

∴ F₁  = μ N₁  = 0.3 × 433 = 129.9 N

Putting this in Eq. (7), we obtain

P₁ = 250 - 129.9 = 120.1 N

Case II

While the body is just on the point of moving up, the frictional force shall be acting downwards.

Figure 3.12

Resolving all of the forces parallel to the inclined plane, we obtain

P₂  - F₂  - W sin 30^o  = 0

∴ P₂  = 500 sin 30^o + F₂  = 250 + F₂

Resolving all of the forces normal to the inclined plane, we obtain

N ₂  - W cos 30^o  = 0

∴ N ₂  = 500 cos 30^o  = 433 N

∴ F₂  = μ N ₂  = 0.3 × 433 = 129.9 N

Putting the value of F2 in Eq. (9), we obtain

P₂  = 250 + 129.9 = 379.9 N

Therefore, it is seen that while the force applied is 379.9 N or more the body shall move up and while it is 120.1 N or less it shall move down. While the force applied is between 120.1 N and 379.9 N, the body shall neither move upwards nor downwards, experiencing variable friction varying from 129.9 N acting upwards reducing to zero and then increasing up to 129.9 N acting downwards.

The mistake most often made in the solution of problems involving friction is to write the frictional force in the form F = μ N. This is to be remembered that only in case of impending or actual sliding motion of bodies with respect to one another the frictional force shall be maximum, i.e. F_max = μ N. In all other cases, the frictional force working on a body is found by solving the equations of static equilibrium of the body.