HEIGHTS AND DISTANCES
If the angle of elevation of cloud from a point 'h' meters above a lake is α and the angle of depression of its reflection in the lake is β, prove that the height of the cloud is.
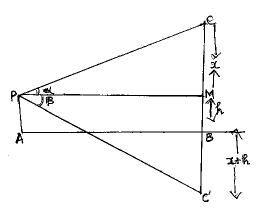
Ans : If the angle of elevation of cloud from a point 'n' meters above a lake is ∝ and the angle of depression of its reflection in the lake is β, prove that the height of the cloud is h
( tan β + tan α / tan β - tan α )
Let AB be the surface of the lake and
Let p be an point of observation such that AP = h meters. Let c be the position of the cloud and c' be its reflection in the lake. Then ∠CPM = ∝ and ∠ MPC1 = β.
Let CM = x.
Then, CB = CM + MB = CM + PA = x + h
In ? CPM, we have tan ∝ = CM /PM
⇒ tan ∝ = x/AB
[∴ PM = AB]
⇒ AB = x cot∝ ...........1
In ? PMC', we have
tanβ = C 'M/PM
⇒ tanβ = x + 2h/AB [Θ C'M=C'B+BM = x + h + n]
⇒ AB = (x + 2h) cot β
From 1 & 2
x cot ∝ = (x + 2h) cot β
x (cot ∝ - cot β) = 2h cot β (on equating the values of AB)
x= ( 1/tan α -1/tan β) = 2h /tan β = x(tan β - tan α/tan α - tan β) = 2h /tan β
x = 2h tan α /tan β - tan α
Hence, the height CB of the cloud is given by CB is given by CB = x + h
⇒ CB= 2h tan α /tan β - tan α + h
2h tan α + h tan β - tan α
⇒ CB- 2h tan α + h tan β - h tan α /tan β - tanα= h (tan α + h tan β )/tan β - tan α